Reviewing the Assessment of Optimal Yield of Groundwater in Korea

1. Introduction

The optimal pumping rate, or optimal groundwater intake is the maximum pumping rate that can be maintained from a target well without an excessive reduction in groundwater level. The optimal yield is the groundwater amount that maintains the dynamic, long term equilibrium state of the groundwater system, within a level that does not exceed the natural recharge rate (Hamm et al., 1998). According to the guidelines for groundwater works (Ministry of Environment, 2020), during the step-drawdown test, the critical yield is set as the pumping rate of the starting water level, from which it rapidly declines, and the optimal yield is determined within the range that does not exceed the critical yield. The critical yield is the maximum amount that can be pumped from a well without causing excessive drawdown, and rapid drawdown occurs just over the critical yield. Therefore, the optimal yield can be defined as the amount that considers both the safety factor and critical yield. The optimal yield is determined using a hydrogeological survey.

Safe, sustainable, and optimal yield refer to the amount of groundwater used within a groundwater basin that will not cause depletion of groundwater resources or inflow of pollutants in the long term (Domenico et al., 1968). Lee(1915) defined the safe annual yield as the amount of groundwater that may be extracted permanently and consistently without the risk of aquifer depletion. Thomas(1951) and Kazmann(1956) argued that the definition of optimal pumping amount was insufficient, as in regions with high inflow from a river to an aquifer, no upper limit for the optimal pumping amount may exist. Mann(1963) indicated that the optimal pumping amount can easily be misunderstood in terms of laws.

In this study, we examined the current problems, improvements, and methods for optimal groundwater yield estimation in Korea while considering sustainable groundwater development.

2. Materials and Method

2.1. Methods of estimating optimal pumping rate

The optimal yield is estimated both for individual wells and for the entire groundwater basin. Generally, the optimal yield for individual wells can be determined using long-term pumping and step drawdown tests. The step-drawdown test is widely used internationally (Cho et al., 2017). Several studies have suggested methods to complement this approach. For example, Sharp(2016) described the Hill, Harding, and zero water-level change methods. The Hill method (Conkling, 1946) determines the optimal pumping rate using the annual pumping rate when the average annual change in groundwater level is zero (Fig. 1). The Harding method (Harding, 1927) estimated the optimal yield for a shallow phreatic aquifer by subtracting the outflow (pumping amount, spring outflow, and baseflow) from the inflow of the aquifer (recharge amount, river inflow, and irrigation return flow) corresponding to an average annual groundwater level change of zero. The zero-groundwater-level change method evaluates the optimal yield as the average pumping amount during the time interval between two periods when the groundwater level is the same.

Fig. 1.

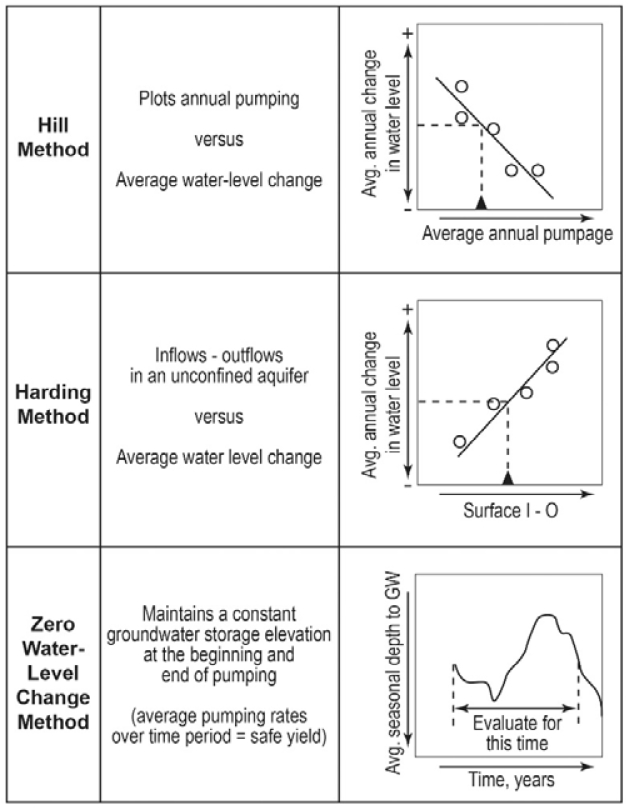
Optimal yield estimation by using the methods of Hill, Harding, and zero groundwater-level from Sharp(2016).

The optimal yield determined in the early stages of groundwater development serves as the standard for groundwater use approval. However, the initially determined optimal yield can change due to natural or artificial factors as well as increased groundwater development in a groundwater basin. Depending on natural factors, the optimal yield is changed by an increase or decrease in the infiltration rate and groundwater recharge rate as well as in precipitation. In addition, the optimal yield is influenced by artificial factors, for example by increasing the pumped water in the groundwater basin. Alternatively, when the number of wells or the total pumping rate increases in the groundwater basin, the optimal pumping amount decreases due to the effects of the interference and superposition of the wells.

3. Results

3.1. Optimal yield estimation by step-drawdown test

Using the step-drawdown test method, the critical yield is first derived and subsequently the optimal yield is estimated by considering the safety factor within the range of the critical yield (Cho et al., 2017; Ministry of Environment, 2020). The safety factor can be determined by assessing the water level decline relative to the aquifer size and recharge rate. The safety factor for the optimal yield is lower for deep-bedrock aquifers than for shallow phreatic aquifers, because the groundwater recharge rate in shallow phreatic aquifers is much greater than that in deep-bedrock aquifers.

In the step-drawdown test, the drawdown from a pumping well is measured at a certain pumping rate until the water level stabilizes, and then the pumping rate is increased or decreased for drawdown measurement. Typically, step-drawdown tests are performed by increasing the pump rate. The pumping rate is changed when the drawdown is stabilized during the step-drawdown test. However, because perfect water level stabilization is uncommon, the pseudo-steady water level condition is considered to be a stable water level. Hence, it is important to decide a drawdown rate that may represent a quasi-equilibrium state. The time interval for a pumping stage is usually over an hour until a quasi-equilibrium state is reached and should be similar to other pumping stages. In addition, when two or more pumping wells are present in the target area, the optimal yield is determined by considering the interference effect between the pumping wells (Ministry of Environment, 2013).

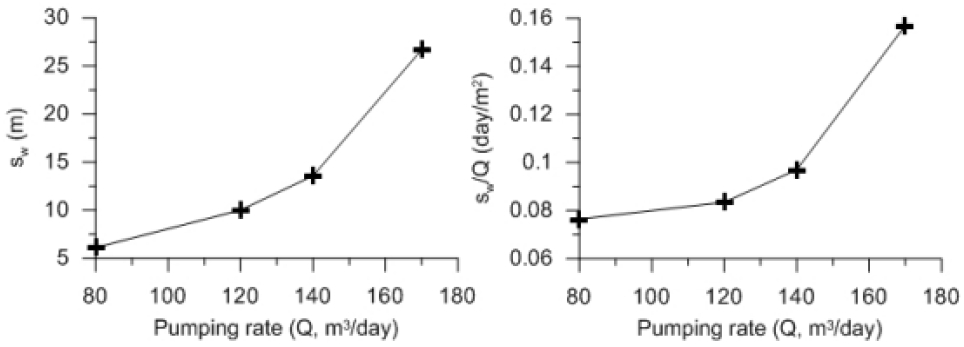
A step-drawdown test is used to determine the critical yield, the well loss and well storage effects, well efficiency, and hydraulic parameters. Lee(2016) stated that the step- drawdown test is not a tool for determining the optimal yield, because the pumping amount by the step-drawdown test includes artificial factors (well loss effect, well storage effect, and well efficiency), which are driven by well construction, not by the hydraulic characteristics of the aquifer. By Lee(2016), the step-drawdown test is not suitable for determining critical yield becuase it is decided by a critical point from the relationship between the increase in the specific drawdown and the increase in the pumping rate (Fig. 2).

Fig. 2.

Example of drawdown (sw) vs. pumping rate (Q) and sw/Q vs. Q from Lee(2016).

3.2. Optimal yield estimation by long-term pumping test

A long-term pumping test (or constant pumping test) measures the drawdown during constant pumping for a long period such as 24 hours (Fig. 3). In general, a long-term pumping test is used to determine the hydraulic parameters (hydraulic conductivity, transmissivity, storage coefficient, specific capacity, etc.) of an aquifer, and to understand its vertical and lateral sizes. While performing this test, a pumping well or both a pumping well and one or more observation wells are utilized. Furthermore, during this test, changes in the groundwater level are measured in the pumping and observation wells. The water level data recorded during the long-term pumping are used to analyze the pumping test. Through the pumping test analysis, the hydraulic parameters and characteristics and dimensional size of the aquifer are determined; subsequently, the optimal yield with the corresponding drawdown is determined.

Fig. 3.

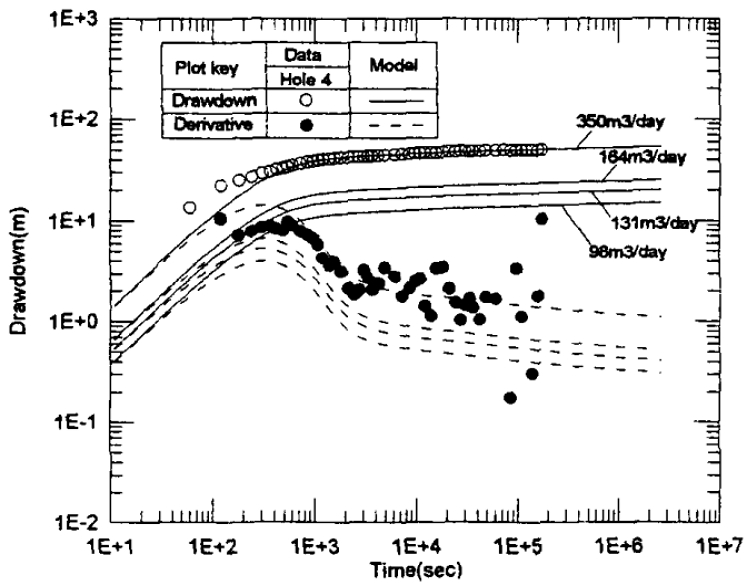
Example of determining the optimal pumping amount by long-term pumping test of bedrock fractured aquifer from Hamm et al.(1998).

Long-term pumping tests have the advantage of determining the optimal yield which is suitable for different aquifer types (unconfined, confined, leaky confined, and dual-porosity aquifers) (Fig. 3). In this case, plotting drawdown rate versus the elapsed pumping time together with the drawdown curve facilitates the identification of the type and dimensions of the aquifer (Bourdet et al., 1983). Moreover, careful attention should be paid to the conditions and assumptions for the pumping test analysis because the analysis is dependent on the aquifer type (unconfined aquifer, confined aquifer, leaky confined aquifer, dual-porosity aquifer, unconsolidated bed, and bedrock), geometry of the aquifer boundary, depth and shape of the well, and well conditions (well storage effect, well loss effect, and interval of the well screen).

Most deep bedrock aquifers are confined, demonstrating that the groundwater recharge rate is slow, with a slow aquifer response. Groundwater reaching the deep bedrock aquifer takes a very long time because it must pass through the shallow phreatic aquifer in the highland or the confining layer of low permeability located above the confined aquifer. Therefore, the groundwater recharge rate in deep bedrock aquifers largely depends on the characteristics of the low-permeability confining layer. In general, a limited recharged amount to the deep bedrock aquifer, passing through underground, mostly comes from a small surface area of shallow phreatic aquifers in the highlands. In addition, highlands have a large topographic gradient, which results in insufficient time for rainfall to infiltrate the ground. Eventually, the groundwater recharge rate in the deep bedrock aquifer may be much lower than that in the shallow aquifer. However, in cases where large-scale permeable fracture zones are well-developed in deep bedrock aquifers, groundwater moves rapidly through the fracture zones under confined conditions. The pumping of groundwater from most deep bedrock aquifers induces a decline in the groundwater level, and once the groundwater level drops, it is unrecoverable, similar to groundwater mining. Hence, in the case of pumping from deep bedrock aquifers, the limit of groundwater decline should be carefully determined. Under these circumstances, the optimal yield can be determined from a cost-benefit perspective (Msangi and Hejazi, 2022).

3.4. Estimation of sustainable yield for the entire groundwater basin

For an entire groundwater basin, the sustainable yield is equal to the pumped amount of groundwater that is maintained for an indefinite time without unacceptable environmental, economic, and social circumstances (Lin and Lin, 2019). To determine the sustainable yield of an entire groundwater basin, identifying the boundary conditions, basin domain, aquifer characterization, and groundwater recharge rate of the basin is necessary. The groundwater basin is bordered by the geometry of geological strata, and accordingly, the surface boundary of the groundwater basin differs from that of the catchment. Sustainable yield relies considerably on the recharge or impervious boundaries of the groundwater basin. The boundary conditions of a groundwater basin can be determined using geological surveys, hydrogeological inventories, geophysical surveys, and hydraulic tests. In particular, the drawdown data obtained from the pumping tests provide a thorough interpretation because they supply useful information on the groundwater flow within the groundwater basin.

To determine the sustainable yield for the entire groundwater basin, it is also necessary to perform groundwater modeling, which can measure sustainable yields by analyzing the groundwater level response relative to various pumping scenarios (Lin and Lin, 2019). The optimal yield of each well in the basin must be determined within the total sustainable yield of the entire groundwater basin, such that the optimal yield of a new well must be less than the remaining total sustainable amount, exempting the total optimal yield of the existing wells. That is, the sum of the total optimal yields in the groundwater basin cannot exceed the sustainable yield of the entire groundwater basin or the natural recharge rate of the groundwater basin, which is governed by precipitation in the basin. In addition, the hydraulic conductivity of the surface layer is closely related to the groundwater recharge rate.

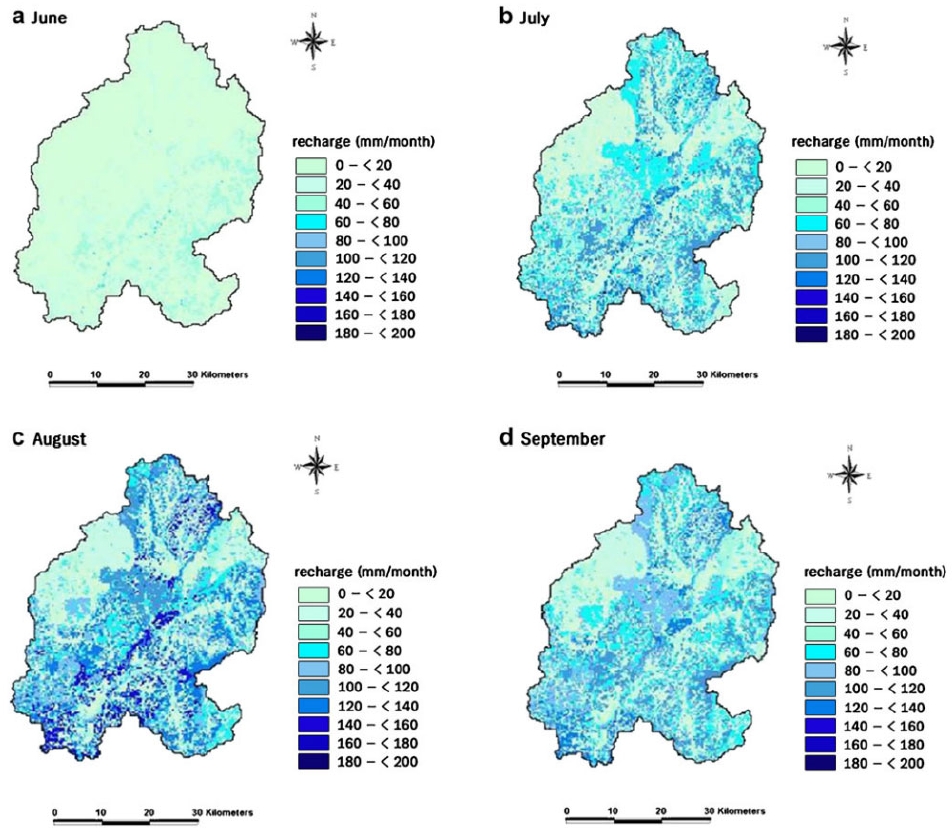
As explained above, an accurate estimation of the groundwater recharge rate is essential for determining a sustainable yield in the groundwater basin. Methods for estimating groundwater recharge rate include the water balance analysis method (Seonwoo, 1992; Hamm et al., 2005), SCS-CN method (Chung and Kim, 2000; Bae and Kim 2006), groundwater level fluctuation method (Healy and Cook, 2002; Moon et al., 2004), groundwater level attenuation curve method for the number of days without rainfall (Lee, 1995; Choi and Ahn, 1998), baseflow separation method (Meyboom, 1961; Park, 1996a, 1996b), environmental isotope method (Andres and Egger, 1985; Solomon et al., 1993; Koh et al., 2001), chloride ion concentration method (Sukhija et al., 1996), and numerical modeling method (Arnold et al., 2000; Chung et al., 2010). Chung et al.(2010) used the SWAT-MODFLOW model (an integrated model of surface water and groundwater) based on water balance components to estimate groundwater recharge (Fig. 4). Among the methods used for groundwater recharge rate estimation, the mass balance equation based on the water cycle is used for water balance, groundwater level fluctuation, groundwater-level attenuation curve, and baseflow separation methods. However, each method has limitations in estimating the groundwater recharge rate, depending on the input parameters. The difference between the groundwater recharge rate in the recharge area and baseflow in the discharge area occurs due to different application methods, such as evapotranspiration at the riverside, groundwater movement into deep groundwater systems, and groundwater outflow to the coast (Chen and Lee, 2003). Currently, the most accurate method among the above-mentioned methods for estimating groundwater recharge rate is unclear. Therefore, many private companies and public institutions in Korea present an averaged value of the groundwater basin recharge rates obtained using various methods; however, a simple averaged value is not reasonable for representing the real groundwater recharge rate.

Fig. 4.

Estimated groundwater recharge rates for June, July, August, and September by Chung et al.(2010).

The groundwater recharge rate varies greatly depending on the aquifer type (phreatic aquifer, confined aquifer, alluvial aquifer, or deep bedrock aquifer). The shallow phreatic aquifer had a much higher groundwater recharge rate than the deep bedrock aquifer. Therefore, identifying the aquifer types in a groundwater basin is necessary. The groundwater recharge rate should be estimated using an appropriate method corresponding to the aquifer type. The groundwater recharge rate is high into phreatic and/or alluvial aquifers, with fast aquifer response whereas the groundwater recharge rate is low into confined and/or bedrock aquifers, with slow aquifer response. Therefore, groundwater mining occurs in confined and deep bedrock aquifers. Once the groundwater level is lowered, it recovers very slowly or never. Hence, for confined and deep bedrock aquifers, a higher safety factor must be applied to determine the optimal or sustainable yield.

4. Discussion

The optimal yield of wells is intimately related to the groundwater recharge rate, which relies on the characteristics of the different types of aquifers. The optimal yield of a confined aquifer is solely related to the total annual pumping quantity, whereas that of an unconfined aquifer corresponds to the annual actual groundwater use minus the return flow. Shallow alluvial aquifers are mostly unconfined. Therefore, the groundwater recharge rate is high with a quick aquifer response because the underground water quickly passes through the shallow unsaturated zone to reach the unconfined aquifer. In the case of lowland aquifers that are mainly shallow or alluvial, the groundwater recharge rate largely depends on the hydraulic conductivity of the alluvial or unconsolidated layer.

Consensus yield refers to an agreed quantity by reaching an agreement through discussion between stakeholders with different opinions, and may vary within a certain range in terms of space and time, according to the interests and knowledge of the stakeholders (Pierce, 2006; Sharp et al., 2008; Dulay, 2011). In reality, even if an optimal yield is determined hydrogeologically, this amount cannot be agreed upon by the stakeholders. In this respect, it is meaningful to derive an agreed pumping amount based on the optimal yield. Similar to the agreed pumping amount, the Environmental Impact Assessment Review Committee for drinking mineral water determines the optimal yield of drinking mineral water by reviewing the environmental impact of the pumping amount, which was estimated through the consultants’ hydrogeological survey.

Worldwide, the most common tool for determining the optimal yield is the step drawdown test (Cho et al., 2017). The optimal yield can be determined by groundwater quantity and quality, economic, and water-use rights factors. First, in terms of quantity factor, optimal yield should not exceed the average long-term groundwater supply in the target area. The groundwater supply is nearly equal to the groundwater recharge derived from precipitation. The groundwater supply is regulated by the size of the aquifer and the groundwater flow from the recharge area to the discharge area. Pumping excess groundwater supply is known as groundwater mining.

From the perspective of groundwater quality, the optimal yield in a target area can be affected by pollutants originating from natural or artificial sources. When groundwater quality has been deteriorated due to contaminant input from pumping, groundwater can no longer be used. Saltwater intrusion can occur in coastal areas when groundwater pumping exceeds the capacity of the aquifer. Additionally, pumping from deep groundwater systems can lead to an inflow of groundwater containing salts or high concentrations of mineral components. Hence, the optimal yield must meet the water quality standards.

From an economic perspective, the optimal yield should be such that the economic benefit of groundwater use is greater than the total cost of groundwater pumping (e.g., pumping facilities, electricity fees, and charges for groundwater use). Groundwater pumping costs typically increase in areas with unconfined aquifers with deep water tables or confined aquifers covered by thick impermeable layers. An exceptional case is groundwater development to compensate for water shortages during drought seasons, regardless of economic benefits. According to the beneficiary payment principle in Article 30-3 of the Groundwater Act, groundwater use charges are imposed on persons who use groundwater or public water resources larger than a certain amount, and are utilized for the conservation and systematic use of groundwater.

For groundwater use rights, the optimal yield must be determined within the range of the pumping amount of a new well, which does not affect existing wells. Therefore, to develop a new well, a groundwater impact assessment must be conducted to confirm that the new pumping will not affect the pumping capacity of existing wells. The groundwater impact assessment survey must assess the impact of new wells on existing wells within a radius of influence. According to Paragraphs 2 and 7 of Article 7 (Permission for Groundwater Development and Use) of the Groundwater Act, a person who wishes to acquire a permit for groundwater development and use in advance must request a groundwater impact survey consultant to conduct a groundwater impact survey and submit the survey report to the mayor, county head, or district head, who reviews the survey report and reflects it in the permit contents.

5. Conclusions

The optimal yield includes both the optimal yield of an individual well and the sustainable yield of the entire groundwater basin. The optimal yield is based on variables such as quantity, quality, economic benefit, and water use rights factors, as well as the agreement between stakeholders. The basic method for calculating the optimal yield is to use step-drawdown and long-term pumping tests. Hence, the optimal yield can be determined by analyzing the long-term pumping and step-drawdown tests that must be conducted when developing a new well. In the step drawdown test, the critical yield is determined and then the optimal yield is determined by considering the safety factor. The safety factor can be decided by the percentage of drawdown relative to the aquifer size which will be allowed, with considering the groundwater recharge rate in the target area. Thereafter, the groundwater-level decline observed in the step-drawdown test is reviewed using the hydraulic parameters obtained from the long-term pumping test. Finally, the optimal yield is determined by considering both the long-term groundwater decline and the safety factor that can be corrected by the natural recharge rate in the target area. Groundwater level decline caused by long-term pumping over many years may be simulated and predicted through numerical modeling. The natural recharge rate for the numerical model may be presented by the injection rate into the well.

The optimal yield of an individual well is closely related to the sustainable yield of the groundwater basin, which can be assessed through a basic hydrogeological survey and be determined by groundwater modeling using data obtained from the hydrogeological survey in the groundwater basin. Because the sum of the optimal yields of all wells within the basin cannot exceed the sustainable yield of the entire groundwater basin, the optimal yield of a new well must be determined by considering the optimal yield of the existing wells. The sustainable yield of the entire groundwater basin must be lower than the groundwater recharge rate, which is governed by precipitation. The groundwater recharge rate in the groundwater basin must be estimated using suitable methods that comply with the aquifer types in the basin, which must be classified in advance. In addition, the infiltration rate of the surface layer is directly linked to the rate and amount of groundwater recharge.

The optimal yield of individual wells needs to be re-estimated periodically with the groundwater level decline, similar to the sustainable yield of the entire groundwater basin. Re-estimation of the optimal yield may be performed every five years, as in the case of drinking mineral water. When re-estimating the optimal yield, the groundwater recharge rate, hydraulic interference from nearby wells, influence of potential pollutants, and radius of influence must be considered. In particular, when executing hydrogeological surveys or groundwater impact assessment surveys, recalculation of not only the optimal yield of the target well, but also that of the surrounding wells within a certain radius (e.g., 1-km radius) is essential. In addition, seasonal effects should be considered when estimating the optimal yield, because the optimal yield may be affected by seasonal precipitation. Hence, it may be reasonable to determine the optimal yield based on the estimated optimal yield at least twice per year. Meanwhile, if the groundwater level and pumping quantity data are available for at least one year, the optimal yield can be re-estimated using the Hill, Harding, and zero groundwater-level change methods.

REFERENCES

• Andres, G., Egger, R., 1985, A New tritium interface method for determining the recharge rate of deep groundwater in the Bavarian Molasse basin, J. Hydrol., 82, 27-38. [https://doi.org/10.1016/0022-1694(85)90044-7]
• Arnold, J. G., Muttiah, R. S., Srinivasan, R., Allen, P. M., 2000, Regional estimation of base flow and groundwater recharge in the upper Mississippi river basin, J. Hydrol., 227, 21-40. [https://doi.org/10.1016/S0022-1694(99)00139-0]
• Bae, S. K., Kim, Y. H., 2006, Estimation of groundwater recharge rate using the NRSC-CN and the baseflow separation methods, J. Environ. Sci., 15, 253-260. [https://doi.org/10.5322/JES.2006.15.3.253]
• Bourdet, D., Whittle, T. M., Douglas, A. A., Pirard, Y. M., 1983, A New set of type curves simplifies well test analysis, World Oil, 95-106.
• Chen, W. P., Lee, C. H., 2003, Estimating ground-water recharge from streamflow records, Environ. Earth Sci., 44, 257-265. [https://doi.org/10.1007/s00254-002-0753-2]
• Cho, B. W., Yun, U., Moon, S. H., Lee, B. D., Cho, S. Y., Kim, Y. C., Shin, J. H., Hwang, S. H., Ha, K. C., 2017, Optimal pumping rate of a water well at lmgokri, Sangju city, The J. Eng. Geol., 27, 255-265.
• Choi, B. S., Ahn, J. G., 1998, A Study on the estimation of regional groundwater recharge ratio, J. the Korean Soc. Groundwater Environ., 5, 57-65.
• Chung, Y. H., Kim, K., H., 2000, Estimation of groundwater recharge rate with SCS-CN method in Miwon, J. Inst. of Construction Tech., 19, 181-190.
• Conkling, H., 1946, Utilization of ground-water storage in stream system development, Trans. Amer. Soc. Civil Engineers, 111, 275-305. [https://doi.org/10.1061/TACEAT.0005914]
• Chung, I. M., Kim, N.-W., Lee, J., Sophocleous, M., 2010, Assessing distributed groundwater recharge rate using integrated surface water-groundwater modelling: Application to Mihocheon watershed, South Korea, Hydrogeology J., 18, 1253. [https://doi.org/10.1007/s10040-010-0593-1]
• Domenico, P. A., Anderson, D. V., Case, C. M., 1968, Optimal ground‐water mining, Water Resour. Res., 4, 247-255. [https://doi.org/10.1029/WR004i002p00247]
• Dulay, M. P., 2011, From Chaos to Harmony: Public Participation and Environmental Policy, Ph.D. dissertation, University of Texas, Austin, Texas.
• Hamm, S. Y., Lim, J. U., Bae, D. J., Chwae, U. C., 1998, A Study on the determination of optimal yield from fissured aquifers, J. the Korean Soc. Groundwater Environ., 5, 21-29.
• Hamm, S. Y., Cheong, J. Y., Kim, H. S., Hahn, J. S., Cha, Y. H., 2005, Groundwater flow modeling in a riverbank filtration area, Desan-Myeon, Changwon City, Economic and Environ. Geol., 38, 67-78.
• Harding, S. T., 1927, Ground water resources of southern San Joaquin valley: Bull., Div. of Eng. and Irrig, Dept. of Public Works, State of California, 11.
• Healy, R. W., Cook, P. G., 2002, Using groundwater levels to estimate recharge, Hydrogeology J.,10, 91-109. [https://doi.org/10.1007/s10040-001-0178-0]
• Kazmann, R. G., 1956, "Safe yield" in ground-water development, reality or illusion?, Proc. Amer. Soc. Civil Engineers, 82(IR3), 1-12. [https://doi.org/10.1061/JRCEA4.0000013]
• Koh, Y. K., Bae, D. S., Kim, C. S., Kim, K. S., Chung, H. J., Kim, S. Y., 2001, Consideration of the groundwater recharge based on environmental isotopic characteristics of the small basin in the Yeosu area, J. Korean Soc. Soil and Groundwater Environ., 6, 93-106.
• Lee, B. D., Moon, H. S., 2019, Improvement measures of the permit system in natural mineral water, J. Environ. Policy and Administration, 27, 161-187. [https://doi.org/10.15301/jepa.2019.27.4.161]
• Lee, C. H., 1915, The determination of safe yield of underground reservoirs of the closed-basin type, Trans. Amer. Soc. Civil Engineers, 78, 148-251. [https://doi.org/10.1061/TACEAT.0002653]
• Lee, D. R., 1995, Prediction of 1ong-term 1ow streamflow and groundwater recharge using groundwater recession curve, Ph. D. thesis, Korea University, Seoul, Korea, 359.
• Lee, J. Y., 2016, Step-drawdown test is not a tool to determine the so called optimal discharge rate, J. the Geol. Soc. Korea, 52, 443-446. [https://doi.org/10.14770/jgsk.2016.52.4.443]
• Lin, L., Lin, H., 2019, Determination of groundwater sustainable yield using a numerical modelling approach for the Table Mountain Group sandstone aquifer, Rawsonville, South Africa, Hydrogeology J., 27, 841-855. [https://doi.org/10.1007/s10040-018-1902-3]
• Mann, J. F., Jr., 1963, Factors affecting the safe yield of ground-water basins, Trans. Amer. Soc. Civil Engineers, 128, 180-190. [https://doi.org/10.1061/TACEAT.0008891]
• Meyboom, P., 1961, Estimating groundwater recharge from stream hydrographs, J. Geophys. Res., 66, 203-1214. [https://doi.org/10.1029/JZ066i004p01203]
• Ministry of Environment, 2013, Guidelines of Environmental Impact Survey for Drinking Mineral Water Development, 31.
• Ministry of Environment, 2020, Guidelines for Performance of Groundwater Works, 327.
• Moon, S. K., Woo, N. C., Lee, K. S., 2004, Statistical analysis of hydrographs and water-table fluctuation to estimate groundwater recharge, J. Hydrol., 292, 198-209. [https://doi.org/10.1016/j.jhydrol.2003.12.030]
• Msangi, S., Hejazi, M., 2022, How stable is the stabilization value of groundwater? Examining the behavioral and physical determinants, Water Resour. and Economics, 38, 100195. [https://doi.org/10.1016/j.wre.2022.100195]
• Park, C. K., 1996a, Estimation of the available amount of groundwater in South Korea: 1. Development of the method, J. the Korean Soc. Groundwater Environ., 3, 15-20.
• Park, C. K., 1996b, Estimation of the available amount of groundwater in South Korea: 2. Application of five major river basins, J. the Korean Soc. Groundwater Environ., 3, 21-26.
• Pierce, S. A., Dulay, M. M., Sharp, J. M., Lowry, T. S., Tidwell, V. C., 2006, Defining tenable groundwater management: Integrating stakeholder preferences, distributed parameter models, and systems dynamics to aid groundwater resource allocation: in MODFLOW and More 2006: Managing Groundwater Systems (Poeter E., Hill, M. & Zheng, C-M., eds.), Golden, Colorado, International Ground Water Modeling, 409-413.
• Sharp, J. M., Jr., 2016, Sustainability of groundwater resources: Conceptual evolution, opportunities, & challenges: in sustaining Ireland’s water future: The role of groundwater, Proceedings, 36th Annual Groundwater Conference, Inter. Assoc. Hydrogeologists, Irish Chapter, Tullamore, Ireland, Session 1, 11. [https://doi.org/10.1130/abs/2017SC-288962]
• Sharp, J. M., Jr., Pierce, S. A. Smith, B. A., Dulay, M. P., Eaton, D. J., 2008, Conflict resolution and integration of science in groundwater policy development, Water Down Under 2008, 2453-2462.
• Solomon, D. K., Schief, S. L., Poreda, R. J., Clarke, W. B., 1993, A Validation of ³H/³He method for determining groundwater recharge, Water Resour. Res., 29, 2951-2962. [https://doi.org/10.1029/93WR00968]
• Sukhija, B. S., Reddy, D. V., Nagabhushanam, P., Hussain, S., 1996, Environmental and injected tracers methodology to estimate direct precipitation recharge to a confined aquifer, J. Hydrol., 177, 77-97. [https://doi.org/10.1016/0022-1694(95)02788-2]
• Sunwoo, J.-H., 1992, Necessity of groundwater development in Korea, J. Korea Water Resour. Assoc., 25, 6-17.
• Thomas, H. E., 1951, The Conservation of Groundwater, McGraw-Hill, New York.